Photoionization Theory for Coherent and Incoherent Light

In the following, the semi-classical approach for photoionization formulated in my paper Scattering of Radio Waves by High Atomic Rydberg States is developed further such as to be applicable not only for coherent light but also for light which is not sufficiently coherent in order to ionize an atom within the coherence time. In the paper, the latter case was (although not being significant for the results) taken into account by applying a corresponding reduction factor to the photoionization cross section, but this was somewhat of an ad-hoc procedure and not consistently derived from an appropriate interaction model. On this page, the 'low coherency' case is instead being developed in a straightforward manner from the coherent case by assuming the photoionization to be due to a stepwise accumulation of (pseudo)-energy before the actual transition from the ground state into the continuum can take place.

Coherent Photo Ionization

The interaction of light with atoms can generally be described as a forced oscillator (in the case of photoionization as a Pseudo-Oscillator). If the oscillation is in phase with the driving field of the electromagnetic wave throughout, this is exactly equivalent to the acceleration of an electron by a constant electric field E (where E is the amplitude of the wave). The electron will therefore be accelerated to the velocity v within a time

(1) T_c=v/a = v/(eE/m) ,

where e is the elementary charge.
Since v is related to the energy ε by

(2) v= √(2ε/m) (m=electron mass) ,

and ε has to be taken as identical to hν (h=Planck-constant, ν=wave-frequency), this yields

(3) T_c= √(2hν^.m) /(eE) .

After evaluating the constants, this gives the numerical expression

(4) T_c= 7^.10^-18^.√ν /E [sec] (in Gausssian cgs-units i.e. ν [Hz], E [statvolt/cm](=3^.10⁴V/m) ) .

For sunlight (assuming E=10^-2 statvolt/cm), this amounts to about 10^-8 sec, i.e. the almost instantaneous release of electrons in the photoeffect can well be explained within the wave theory of light if a proper interaction model is used (of course, the wave frequency ν has to be high enough here to enable photoionization in the first place)(note: the assumption E=10^-2 statvolt/cm should be merely considered to be exemplary here as the electric field strength E of the radiation field is in fact unknown; it has been derived here by equating the well known 'energy' flux of the sun with c^.E²/4π , which however (as pointed out in the introduction to my page Wave and Particle Theory of Light applied to the Photoelectric Effect on my site physicsmyths.org.uk), is theoretically flawed, and ambiguous anyway due other physical parameters affecting the measured intensity as addressed on this page).

However, it is obvious that only if the acceleration is uniform, is the actual ionization time equal to T_c. This requires that T_c is short compared to the coherence time τ_c of the electromagnetic wave or the time between particle collisions disturbing the ionization process. Otherwise one has to modify the argument (see below).

Incoherent Photo Ionization

If the time T_c required to ionize the atom is not shorter than the coherence time of the electromagnetic wave or the time between particle collisions disturbing the ionization process, the above consideration can not be applied anymore as the acceleration of the electron can not be uniform due to phase jumps occurring. In this case the necessary ionization energy can only be reached in a stepwise manner. If τ_c is the coherence time of the wave field, then the associated increase in velocity Δv during this time interval is

(5) Δv = (eE/m)^.τ_c .

Associating the related energy increase in a correspondence like fashion by

(6) Δε = m/2^.(Δv)² = (τ_c^.eE)²/(2m) ,

the total time required for incoherent photoionization is then

(7) T_i = τ_c^.hν/Δε = 2hν^.m/(eE)²/τ_c ,

and after evaluating the constants

(8) T_i= 5^.10^-35^.ν /E²/τ_c [sec] (in Gausssian cgs-units i.e. ν [Hz], E [statvolt/cm](=3^.10⁴V/m) ) .

In general, Δε and thus T_i will of course not have a fixed value but merely represent statistical averages as both E and τ_c will show statistical variations.

Applied to the sunlight example, the incoherent photoionization still yields ionization times of the order of 10^-4 sec (assuming a coherence time τ_c=10^-12 sec (this value is based on the coherence length of an individual atomic emission in the solar photosphere (which is determined by the electronic collision rate), and this is also assumed to be the coherence time for the total radiation field; this assumption is supported by corresponding numerical computations (see Coherence Length of Wave Field Formed by Superposition))) .

It is important to note however that T_i should, in contrast to T_c, in general merely be considered as the time required to establish a statistical equilibrium situation rather than the time required for a particular light pulse to release a photoelectron. The point is that in the course of the interaction with the sequence of coherent light pulses with frequency ν, the energy of the (pseudo-) oscillator increases stepwise close to the threshold value h^.ν, and it will then just take one further light pulse of duration <τ_c to ionize the atom and release the photoelectron (at which point the energy h^.ν-ε_Ion (where ε_Ion is the ionization energy) is turned into the kinetic energy of the photoelectron) (see the schematic diagram below) .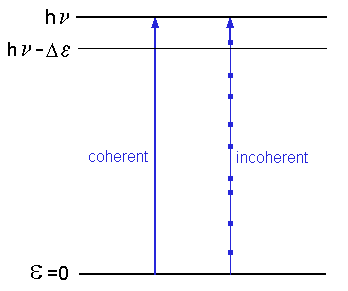

Schematic illustration of photoionization by coherent and incoherent light

Due to the circumstance that in this way the electrons can be brought to a 'pre-ionized' state close to the threshold energy hν by sufficiently incoherent radiation, the apparent 'reaction' time between arrival of the light pulse and release of the photoelectron can in general actually appear to be even faster than for the case of coherent photoionization (if τ_c<T_c). This 'seeding' of the photoionization process could well explain measurements that have been made in the past (e.g. by Lawrence and Beams) which show the release of photoelectrons to be instantaneous to within a few nanoseconds (for presumably very small light intensities).
One should also note that, although the incoherency of light leads to a reduced amount of photoionization, the electromagnetic wave field should still be fully absorbed in the process as it is still doing (pseudo)-work on the atomic electron.

In any case, the apparent intensity of the observed object will be inversely proportional to either T_c or T_i (dependent on whether the light has to be considered as coherent or incoherent), because a faster ionization enables obviously more electrons to be ionized within a given time. For incoherent light, this would therefore recover the E² dependence of the intensity of light in classical electrodynamics mentioned at the beginning. The dependence on the coherence time of the light τ_c in this case also means that the radiation would not produce any ionization at all if τ_c=0 as T_i=∞ then. This circumstance could for instance well resolve 'Olbers' Paradox' for a steady state universe.

One has to bear in mind however that the field strength E is not directly known in most cases but is in fact derived from the photoionization rate over the classical relationship mentioned above (see the paragraph below Eq.(4)). This means that any reduction of the photoionization rate due to incoherency will already (wrongly) be interpreted as a corresponding reduction of the field strength E. In these cases one has to use consequently the formula for the coherent photoionization, as otherwise the reduction would effectively be applied twice (see also my paper about Scattering of Radio Waves by High Atomic Rydberg States (Chpt. 2.4) where the theory for the coherent photoionization was formulated in the first place).